纤维缠绕是制造格栅(geodesic)增强复合材料结构(正交格栅和等边三角形格栅结构壁板)的理想方法,它可使结构的质量很轻,而强度刚度却非常高[1]。
复合材料网格结构在国内应用于工程实践时间尚短,但自动化缠绕成型趋势已十分明显。自动化缠绕成型可提高产品质量、提高生产效率,便于批量生产。
圆柱段网格加筋结构,除纵筋沿母线、环筋沿圆环外,其余筋的中心线均为圆柱螺旋线。截圆锥段网格加筋结构,除纵筋沿母线、环筋为圆环外,其余筋的中心线均为圆锥螺旋线。蒙皮的缠绕除90°沿圆环外,其余缠绕角度均沿圆柱圆锥侧面上的短程线缠绕。因此应对圆柱圆锥侧面上的螺旋线和短程线的几何性质进行深入系统的研究,得出工程上可行的方法以应用于圆柱段和截圆锥段网格加筋结构的几何建模、结构承力计算、自动缠绕时缠绕编程,为复合材料网格结构自动化制造创造条件。本文主要介绍了锥柱面螺旋线短程线与网格缠绕成型技术。
1 圆柱锥侧面上的螺旋线与复合材料网格结构的筋条缠绕成型圆柱段复合材料网格结构,除纵筋和环筋外,其余筋的中心线均为圆柱螺旋线,其轨迹方程为:
| $ \left\{ \begin{array}{l} x = r\cos \theta \\ y = r\sin \theta \\ z = r\cot \alpha \cdot \theta \end{array} \right. $ | (1) |
式中,α为螺旋角。因螺旋角α为定值,当θ=2π为时,z为定值,即圆柱螺旋线是等螺旋角等螺距螺旋线。
圆柱螺旋线方程(1)的切线方程为:
| $ \left\{ \begin{array}{l} \frac{{{\rm{d}}x}}{{{\rm{d}}\theta }} = - r\sin \theta \\ \frac{{{\rm{d}}y}}{{{\rm{d}}\theta }} = r\cos \theta \\ \frac{{{\rm{d}}z}}{{{\rm{d}}\theta }} = r\cot \alpha \end{array} \right. $ | (2) |
设圆柱螺旋线的切线与z轴的夹角为ω,则:
| $ \begin{array}{*{20}{c}} {\cos \omega = \frac{{\frac{{{\rm{d}}z}}{{{\rm{d}}\theta }}}}{{\sqrt {{{\left( {\frac{{{\rm{d}}x}}{{{\rm{d}}\theta }}} \right)}^2} + {{\left( {\frac{{{\rm{d}}y}}{{{\rm{d}}\theta }}} \right)}^2} + {{\left( {\frac{{{\rm{d}}z}}{{{\rm{d}}\theta }}} \right)}^2}} }}}\\ { = \frac{{r\cot \alpha }}{{\sqrt {{{\left( { - r\sin \theta } \right)}^2} + {{\left( {r\cos \theta } \right)}^2} + {{\left( {r\cot \alpha } \right)}^2}} }} = \cos \alpha } \end{array} $ | (3) |
由于圆柱的母线与z轴平行,圆柱螺旋线的切线与z轴的夹角就是圆柱螺旋线的切线与圆柱母线的夹角。圆柱螺旋线的切线与母线的夹角ω与其螺旋角α相等且为定值。
截圆锥段网格加筋结构,除纵筋和环筋外,其余筋的中心线均为圆锥螺旋线。
将螺旋角设为定值,得到等螺旋角圆锥螺旋线轨迹方程[2]:
| $ \left\{ \begin{array}{l} x = \rho \sin \beta cos\theta \\ y = \rho \sin \beta \sin \theta \\ z = \rho \cos \beta \\ \rho = {\rho _0}{{\rm{e}}^{\left( {\frac{{\sin \beta }}{{\tan \alpha }}\theta } \right)}} \end{array} \right. $ | (4) |
式中,β为半锥角。ρ0为坐标原点到截圆锥体小端端面的圆锥侧面上的母线长度。因方程中含有指数函数,等螺旋角的圆锥螺旋筋槽对加工设备要求较高。
将螺距设为定值,得到等螺距圆锥螺旋线轨迹方程[3]:
| $ \left\{ \begin{array}{l} x = a\theta \sin \beta \cos \theta \\ y = a\theta \sin \beta \sin \theta \\ z = a\theta \cos \beta \end{array} \right. $ | (5) |
螺距h=2πacosβ
曲线切线与锥面母线之间夹角的余弦:
| $ \cos \omega = \frac{1}{{\sqrt {1 + {\theta ^2}{{\sin }^2}\beta } }} $ |
式中,aθ为坐标原点到截面锥体小端端面的圆锥侧面上的母线长度。等螺距圆锥螺旋筋槽对加工设备要求较低。模具等速旋转,刀具等速沿圆锥侧面母线运动,即可加工出等螺距圆锥螺旋线。
等螺旋角和等螺距螺旋线在圆锥侧面上是两种曲线,在圆柱侧面上是同一种圆柱螺旋线。
为便于圆柱段和圆锥段复合材料网格结构硬模成型装置的设计生产和纤维缠绕,需要求出圆柱和锥段螺旋线的弧长和曲率。
圆柱螺旋线的弧长为:
| $ \begin{array}{l} S = \int_{{\theta _1}}^{{\theta _2}} {\sqrt {{{\left( {\frac{{{\rm{d}}x}}{{{\rm{d}}\theta }}} \right)}^2} + {{\left( {\frac{{{\rm{d}}y}}{{{\rm{d}}\theta }}} \right)}^2} + {{\left( {\frac{{{\rm{d}}z}}{{{\rm{d}}\theta }}} \right)}^2}} {\rm{d}}\theta } \\ \;\;\;\; = \int_{{\theta _1}}^{{\theta _2}} {r\csc \alpha {\rm{d}}\theta } = r\csc \alpha \left( {{\theta _2} - {\theta _1}} \right) \end{array} $ | (6) |
等螺旋角圆锥螺旋线的弧长为:
| $ \left\{ \begin{array}{l} \frac{{{\rm{d}}x}}{{{\rm{d}}\theta }} = {\rho _0}{{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}\theta }}\frac{{{{\sin }^2}\beta }}{{\tan \alpha }}\cos \theta - {\rho _0}{{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}\theta }}\sin \beta \sin \theta \\ \frac{{{\rm{d}}y}}{{{\rm{d}}\theta }} = {\rho _0}{{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}\theta }}\frac{{{{\sin }^2}\beta }}{{\tan \alpha }}\sin \theta - {\rho _0}{{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}\theta }}\sin \beta \sin \theta \\ \frac{{{\rm{d}}z}}{{{\rm{d}}\theta }} = {\rho _0}{{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}\theta }}\frac{{\sin \beta }}{{\tan \alpha }}\cos \beta \end{array} \right. $ | (7) |
| $ \begin{array}{l} S = \int_{{\theta _1}}^{{\theta _2}} {{\rho _0}\frac{{\sin \beta }}{{\sin \alpha }}{{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}\theta }}{\rm{d}}\theta } = {\rho _0}\frac{{\sin \beta }}{{\sin \alpha }} \cdot \frac{{\tan \alpha }}{{\sin \beta }}{{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}\theta }}/_{{\theta _1}}^{{\theta _2}}\\ = \frac{{{\rho _0}}}{{\cos \alpha }}\left[ {{{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}{\theta _2}}} - {{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}{\theta _1}}}} \right] \end{array} $ | (8) |
等螺距圆锥螺旋线的弧长为:
| $ \left\{ \begin{array}{l} \frac{{{\rm{d}}x}}{{{\rm{d}}\theta }} = a\sin \beta \cos \theta - a\theta \sin \beta \sin \theta \\ \frac{{{\rm{d}}y}}{{{\rm{d}}\theta }} = a\sin \beta \sin \theta + a\theta \sin \beta \cos \theta \\ \frac{{{\rm{d}}z}}{{{\rm{d}}\theta }} = a\cos \beta \end{array} \right. $ | (9) |
| $ S = \int_{{\theta _1}}^{{\theta _2}} {a\sqrt {1 + {\theta ^2}{{\sin }^2}\beta } {\rm{d}}\theta } $ | (10) |
做双曲代换θsinβ=sht,
| $ \begin{array}{*{20}{c}} {S = \frac{a}{{\sin \beta }}\int_{{\rm{arsh}}\left( {{\theta _1}\sin \beta } \right)}^{{\rm{arsh}}\left( {{\theta _2}\sin \beta } \right)} {{\rm{c}}{{\rm{h}}^2}t{\rm{d}}t} }\\ { = \frac{a}{{\sin \beta }}\int_{{\rm{arsh}}\left( {{\theta _1}\sin \beta } \right)}^{{\rm{arsh}}\left( {{\theta _2}\sin \beta } \right)} {\frac{{{\rm{ch2}}t + 1}}{2}{\rm{d}}t} }\\ { = \frac{a}{{2\sin \beta }}\left( {\frac{{{\rm{sh}}2t}}{2} + t} \right)/_{{\rm{arsh}}\left( {{\theta _1}\sin \beta } \right)}^{{\rm{arsh}}\left( {{\theta _2}\sin \beta } \right)}}\\ { = \frac{a}{{2\sin \beta }}\left( {{\rm{sh}}t{\rm{ch}}t + t} \right)/_{{\rm{arsh}}\left( {{\theta _1}\sin \beta } \right)}^{{\rm{arsh}}\left( {{\theta _2}\sin \beta } \right)}}\\ { = \frac{a}{{2\sin \beta }}\left( {{\theta _2}\sin \beta \sqrt {\theta _2^2{{\sin }^2}\beta + 1} - {\theta _1}\sin \beta \sqrt {\theta _1^2{{\sin }^2}\beta + 1} + } \right.}\\ {\left. {{\rm{arsh}}\left( {{\theta _2}\sin \beta } \right) - {\rm{arsh}}\left( {{\theta _1}\sin \beta } \right)} \right)} \end{array} $ | (11) |
圆柱螺旋线:
| $ \left\{ \begin{array}{l} \frac{{{{\rm{d}}^2}x}}{{{\rm{d}}{\theta ^2}}} = - r\cos \theta \\ \frac{{{{\rm{d}}^2}y}}{{{\rm{d}}{\theta ^2}}} = - r\sin \theta \\ \frac{{{{\rm{d}}^2}z}}{{{\rm{d}}{\theta ^2}}} = 0 \end{array} \right. $ | (12) |
曲率[2]:
| $ \begin{array}{l} K = \sqrt {\frac{{\left[ {{{\left( {\frac{{{\rm{d}}x}}{{{\rm{d}}\theta }}} \right)}^2} + {{\left( {\frac{{{\rm{d}}y}}{{{\rm{d}}\theta }}} \right)}^2} + {{\left( {\frac{{{\rm{d}}z}}{{{\rm{d}}\theta }}} \right)}^2}} \right]\left[ {{{\left( {\frac{{{{\rm{d}}^2}x}}{{{\rm{d}}{\theta ^2}}}} \right)}^2} + {{\left( {\frac{{{{\rm{d}}^2}y}}{{{\rm{d}}{\theta ^2}}}} \right)}^2} + {{\left( {\frac{{{{\rm{d}}^2}z}}{{{\rm{d}}{\theta ^2}}}} \right)}^2}} \right] - \left( {\frac{{{\rm{d}}x}}{{{\rm{d}}\theta }} \cdot \frac{{{{\rm{d}}^2}x}}{{{\rm{d}}{\theta ^2}}} + \frac{{{\rm{d}}y}}{{{\rm{d}}\theta }} \cdot \frac{{{{\rm{d}}^2}y}}{{{\rm{d}}{\theta ^2}}} + \frac{{{\rm{d}}z}}{{{\rm{d}}\theta }} \cdot \frac{{{{\rm{d}}^2}z}}{{{\rm{d}}{\theta ^2}}}} \right)}}{{{{\left[ {{{\left( {\frac{{{\rm{d}}x}}{{{\rm{d}}\theta }}} \right)}^2} + {{\left( {\frac{{{\rm{d}}y}}{{{\rm{d}}\theta }}} \right)}^2} + {{\left( {\frac{{{\rm{d}}z}}{{{\rm{d}}\theta }}} \right)}^2}} \right]}^3}}}} \\ = \frac{{{{\sin }^2}\alpha }}{r} \end{array} $ | (13) |
即圆柱螺旋线曲率仅与螺旋角和圆柱半径有关,α=0°时为母线,曲率为0,对应复合材料网格结构纵筋;α=90°时曲率最大,对应环筋。
等螺旋角圆锥螺旋线
| $ \left\{ \begin{array}{l} \frac{{{{\rm{d}}^2}x}}{{{\rm{d}}{\theta ^2}}} = {\rho _0}\sin \beta {{\rm{e}}^{\frac{{\sin \beta }}{{{{\tan }^\alpha }}}\theta }}\left( {\frac{{{{\sin }^2}\beta }}{{{{\tan }^2}\alpha }}\cos \theta - 2\frac{{\sin \beta }}{{\tan \alpha }}\sin \theta - \cos \theta } \right)\\ \frac{{{{\rm{d}}^2}y}}{{{\rm{d}}{\theta ^2}}} = {\rho _0}\sin \beta {{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}\theta }}\left( {\frac{{{{\sin }^2}\beta }}{{{{\tan }^2}\alpha }}\sin \theta + 2\frac{{\sin \beta }}{{\tan \alpha }}\cos \theta - \sin \theta } \right)\\ \frac{{{{\rm{d}}^2}z}}{{{\rm{d}}{\theta ^2}}} = {\rho _0}{{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}\theta }}\frac{{{{\sin }^2}\beta }}{{{{\tan }^2}\alpha }}\cos \beta \end{array} \right. $ | (14) |
其曲率为:
| $ K = \frac{{\cos \alpha }}{{{\rho _0}\tan \alpha \sin \beta {{\rm{e}}^{\frac{{\sin \beta }}{{\tan \alpha }}}}}}\sqrt {{{\sin }^2}\alpha {{\sin }^2}\beta + 2{{\sin }^2}\alpha {{\tan }^4}\alpha {{\sin }^2}\beta + {{\sin }^2}\alpha {{\tan }^4}\alpha - {{\tan }^2}\alpha {{\sin }^2}\beta } $ | (15) |
等螺距圆锥螺旋线
| $ \left\{ \begin{array}{l} \frac{{{{\rm{d}}^2}x}}{{{\rm{d}}{\theta ^2}}} = - 2a\sin \beta \sin \theta - a\theta \sin \beta \cos \theta \\ \frac{{{{\rm{d}}^2}y}}{{{\rm{d}}{\theta ^2}}} = 2a\sin \beta \cos \theta - a\theta \sin \beta \sin \theta \\ \frac{{{{\rm{d}}^2}z}}{{{\rm{d}}{\theta ^2}}} = 0 \end{array} \right. $ | (16) |
其曲率为:
| $ K = \frac{{\sin \beta }}{{a\left( {1 + {\theta ^2}{{\sin }^2}\beta } \right)}}\sqrt {\frac{{4 + 3{\theta ^2}{{\sin }^2}\beta + {\theta ^2} + {\theta ^4}{{\sin }^2}\beta }}{{1 + {\theta ^2}{{\sin }^2}\beta }}} $ | (17) |
筋条的缠绕,由于模具上加工了筋槽,纤维丝束可在存在张力的情况下,缠绕到筋槽内,从而成型各种螺旋角的螺旋筋条。在表面上缠绕纤维最稳妥的方法是沿着两点之间的最短路线进行缠绕[1],即蒙皮的缠绕,纤维丝束在张力作用下应沿短程线缠绕。
2.1 圆柱侧面上的短程线圆柱段蒙皮,除0°和90°外,纤维沿圆柱侧面上的短程线进行缠绕,如图 1所示。
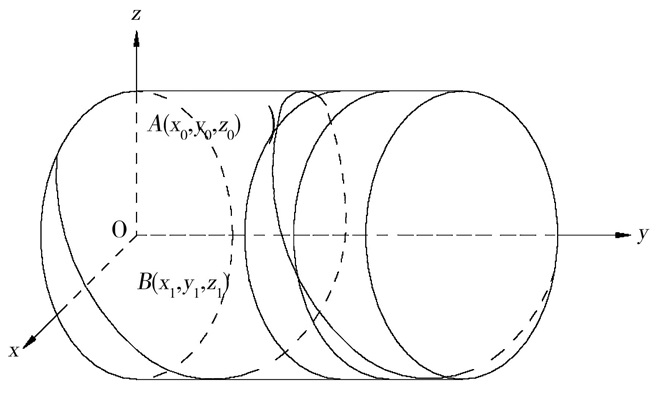
|
| 图 1 圆柱面上的短程线 Figure 1 Geodesicline of column |
设圆柱面方程为:x2+z2=1, (x>0)。并设在圆柱面上连接A(x0, y0, z0), B(x1, y1, z1)两点的曲线方程为:
| $ \left\{ \begin{array}{l} y = y\left( x \right),\\ z = z\left( x \right), \end{array} \right.\left( {{x_0} \le x \le {x_1}} \right) $ |
曲线长度
圆柱面上的短程线问题化为在约束条件
作辅助函数:
| $ I\left[ {y,z} \right] = \int_{{x_0}}^{{x_1}} {\left[ {\sqrt {1 + {{y'}^2} + {{z'}^2}} + \lambda \left( x \right)\left( {z - \sqrt {1 - {x^2}} } \right)} \right]{\rm{d}}x} $ | (18) |
设
| $ H = \sqrt {1 + {{y'}^2} + {{z'}^2}} + \lambda \left( x \right)\left( {z - \sqrt {1 - {x^2}} } \right) $ | (19) |
其欧拉方程组:
| $ \left\{ \begin{array}{l} {H_y} - \frac{{\rm{d}}}{{{\rm{d}}x}}\left( {{H_{y'}}} \right) = 0\\ {H_z} - \frac{{\rm{d}}}{{{\rm{d}}x}}\left( {{H_{z'}}} \right) = 0 \end{array} \right. $ | (20) |
由于H不显含y,欧拉方程组化为:
| $ \left\{ \begin{array}{l} \frac{{\rm{d}}}{{{\rm{d}}x}}\left( {\frac{{y'}}{{\sqrt {1 + {{y'}^2} + {{z'}^2}} }}} \right) = 0\\ \frac{{\rm{d}}}{{{\rm{d}}x}}\left( {\frac{{z'}}{{\sqrt {1 + {{y'}^2} + {{z'}^2}} }}} \right) = \lambda \left( x \right) \end{array} \right. $ | (21) |
求其首次积分,并用弧长s作参数,得:
| $ {\rm{d}}s = \sqrt {1 + {{y'}^2} + {{z'}^2}} {\rm{d}}x $ | (22) |
欧拉方程组化为:
| $ \left\{ \begin{array}{l} \frac{{{\rm{d}}y}}{{{\rm{d}}s}} = {c_1}\\ \frac{{{\rm{d}}z}}{{{\rm{d}}s}} = \omega \left( x \right) \end{array} \right. $ | (23) |
式中,C1为积分常数,以及ω(x)=∫λ(x)dx,
又由
| $ {\rm{d}}x = - \frac{{\sqrt {1 - {x^2}} }}{x}\omega \left( x \right){\rm{d}}s $ | (24) |
根据ds的定义有:
| $ \begin{array}{*{20}{c}} {{{\left( {{\rm{d}}s} \right)}^2} = {{\left( {{\rm{d}}x} \right)}^2} + {{\left( {{\rm{d}}y} \right)}^2} + {{\left( {{\rm{d}}z} \right)}^2}}\\ { = \left[ {\frac{{1 - {x^2}}}{{{x^2}}}\omega \left( x \right) + C_1^2 + {\omega ^2}\left( x \right)} \right]{{\left( {{\rm{d}}s} \right)}^2}} \end{array} $ | (25) |
简化得:
即:
| $ \omega \left( x \right) = \sqrt {1 - C_1^2} x $ | (26) |
带入
| $ - \frac{{{\rm{d}}x}}{{\sqrt {1 - {x^2}} }} = \sqrt {1 - C_1^2} {\rm{d}}s $ | (27) |
积分得:
或:
| $ x = \cos \left( {\sqrt {1 - C_1^2} s + {C_2}} \right) $ | (28) |
于是:
| $ z = \sqrt {1 - {x^2}} = \sin \left( {\sqrt {1 - C_1^2} s + {C_2}} \right) $ | (29) |
再对欧拉方程组第一式积分得:
| $ y = {C_1}s + {C_3} $ | (30) |
综合式(28)、(29)、(30):
| $ \left\{ \begin{array}{l} x = \cos \left( {\sqrt {1 - C_1^2} s + {C_2}} \right)\\ y = {C_1}s + {C_2}\\ z = \sin \left( {\sqrt {1 - C_1^2} s + {C_2}} \right) \end{array} \right. $ | (31) |
由式(1),圆柱侧面上的短程线是其上的螺旋线。
由式(22),用弧长s作参数,弧长s与图 1所示xOz面的极坐标θ及r间的关系为s=cscα·r·θ,为推导方便,已设x2+z2=1, (x>0),即r=1,因此s=cscα·θ, y=cotα·θ。由式(23)第一式,积分常数c1=cosα。由式(28),特定的取积分常数c2为0,事实上当圆柱螺旋线的起点是z轴时,c2=0。
将
得
即
与式(1)相比,这是圆柱半径r=1,以xOz面为圆柱底面向y轴延伸的圆柱螺旋线,而式(1)是圆柱半径为r以xOy面为圆柱底面向z轴延伸的圆柱螺旋线。即式(1)是式(31)的起点在坐标轴的特例。
2.2 圆锥侧面上的短程线及螺旋线的展开圆锥段蒙皮,除0°和90°外,纤维沿圆锥侧面上的短程线进行缠绕, 如图 2所示。设圆锥面方程为:x2+z2=y2tan2θ, (x>0),并设在圆锥面上连接A(x0, y0, z0), B(x1, y1, z1)两点的曲线方程为:
| $ \left\{ \begin{array}{l} y = y\left( x \right),\\ z = z\left( x \right), \end{array} \right.\left( {{x_0} \le x \le {x_1}} \right) $ |
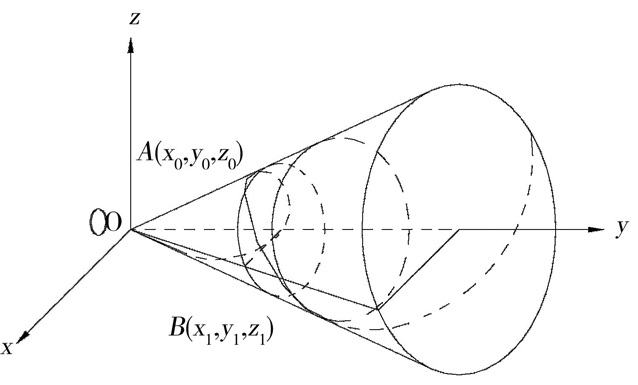
|
| 图 2 圆锥面上的短程线 Figure 2 Geodesicline of cone |
曲线长度:
圆锥面上的短程线问题化为在约束条件
作辅助函数:
| $ \begin{array}{*{20}{c}} {I\left( {y,z} \right) = \int_{{x_0}}^{{x_1}} {\left[ {\sqrt {1 + {{y'}^2} + {{z'}^2}} + \lambda \left( x \right)\left( z \right.} \right.} }\\ {\left. {\left. { - \sqrt {{y^2}{{\tan }^2}\theta - {x^2}} } \right)} \right]{\rm{d}}x} \end{array} $ | (32) |
设
| $ H = \sqrt {1 + {{y'}^2} + {{z'}^2}} + \lambda \left( x \right)\left( {z - \sqrt {{y^2}{{\tan }^2}\theta - {x^2}} } \right) $ | (33) |
其欧拉方程组:
| $ \left\{ \begin{array}{l} {H_y} - \frac{{\rm{d}}}{{{\rm{d}}x}}\left( {{H_{y'}}} \right) = 0\\ {H_z} - \frac{{\rm{d}}}{{{\rm{d}}x}}\left( {{H_{z'}}} \right) = 0 \end{array} \right. $ | (34) |
| $ \left\{ \begin{array}{l} \frac{{\rm{d}}}{{{\rm{d}}x}}\left( {\frac{{y'}}{{\sqrt {1 + {{y'}^2} + {{z'}^2}} }}} \right) = - \frac{{y{{\tan }^2}\theta }}{{\sqrt {{y^2}{{\tan }^2}\theta - {x^2}} }}\lambda \left( x \right)\\ \frac{{\rm{d}}}{{{\rm{d}}x}}\left( {\frac{{z'}}{{\sqrt {1 + {{y'}^2} + {{z'}^2}} }}} \right) = \lambda \left( x \right) \end{array} \right. $ | (35) |
不易分离变量,难以求出短程线方程的解析解。
设有直角三角形纸片的一个锐角为α,将此纸片卷在一圆柱侧面上,使角α的直角边与圆柱的母线重合,角α的斜边则在圆柱面上盘旋上升,这样在圆柱面上就形成一条空间曲线,这条空间曲线就是圆柱螺旋线。上述过程的逆过程,就是将圆柱螺旋线展开,如图 3所示。圆柱面展开其上的圆柱螺旋线是一条直线。能展开的平面,可利用两点之间线段最短来求解。还可以此验证等螺旋角及等螺距圆锥螺旋线是否是圆锥面上的短程线。
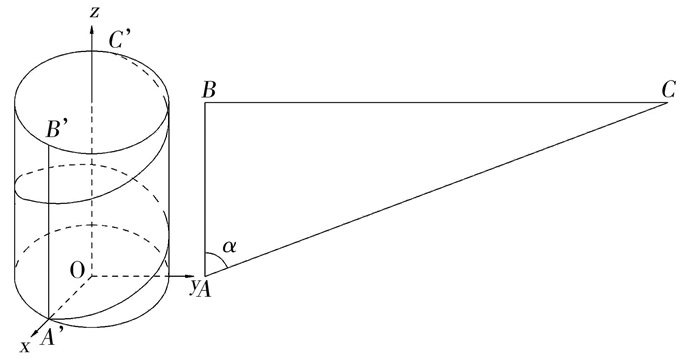
|
| 图 3 圆柱螺旋线的展开 Figure 3 Expansion of the column spiral curve |
另外三角网格的称谓对于圆柱结构是指展开后环筋、纵筋和螺旋筋构成三角形,实际结构三个节点间不会象平面三角形一样稳定。筋条中心线与受力方向夹角越大、曲率越大,越不利于承受对应载荷,即纵筋轴压承载效率高,环筋外压承载效率高,单独轴向直筋承载效率高于螺旋筋。
圆锥侧面展开图如图 4所示。
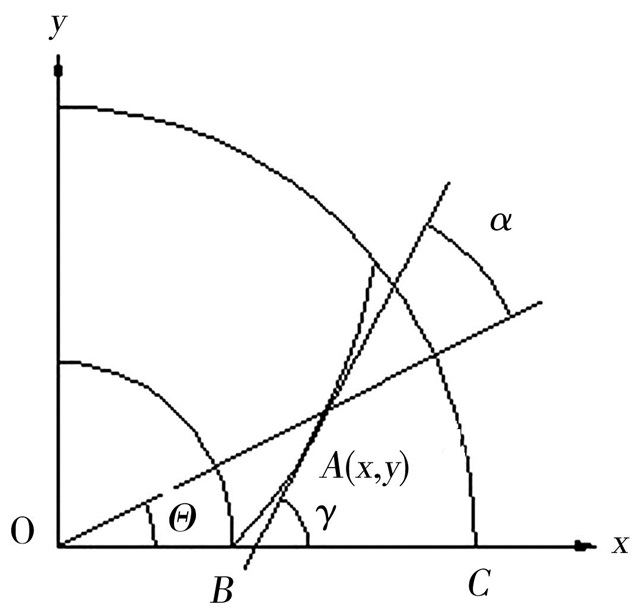
|
| 图 4 圆锥侧面展开图 Figure 4 Spread out side of the cone |
取平面曲线上任意一点A(x,y), 极坐标A(r, Θ),此时曲线的切线与母线的夹角(曲线的切线与切点和极点的连线间的夹角)仍为α。l为截锥壳母线长度,r1为截锥壳上端面半径,r2为截锥壳下端面半径,x0=ρ0=r1/sinβ为B点到O点的距离。x0、r1、r2、l满足关系式:
假设曲线的极坐标方程为:
| $ r = r\left( \mathit{\Theta } \right) $ | (36) |
利用直角坐标与极坐标的关系;x=rcosΘ,
y=rsinΘ则方程组:x=r(Θ)·cosΘ及y=r(Θ)·sinΘ是曲线的参数方程,其中参数为极角Θ,曲线的切线斜率是:
| $ \begin{array}{*{20}{c}} {y' = \frac{{\frac{{{\rm{d}}y}}{{{\rm{d}}\mathit{\Theta }}}}}{{\frac{{{\rm{d}}x}}{{{\rm{d}}\mathit{\Theta }}}}} = \frac{{r'\left( \mathit{\Theta } \right)\sin \mathit{\Theta } + r\left( \mathit{\Theta } \right)\cos \mathit{\Theta }}}{{r'\left( \mathit{\Theta } \right)\cos \mathit{\Theta } - r\left( \mathit{\Theta } \right)\sin \mathit{\Theta }}}}\\ { = \frac{{r'\left( \mathit{\Theta } \right)\tan \mathit{\Theta } + r\left( \mathit{\Theta } \right)}}{{r'\left( \mathit{\Theta } \right) - r\left( \mathit{\Theta } \right)\tan \mathit{\Theta }}}} \end{array} $ | (37) |
根据几何关系可得出公式:
| $ \alpha = \gamma - \mathit{\Theta } $ | (38) |
| $ \tan \alpha = \tan \left( {\gamma - \mathit{\Theta }} \right) = \frac{{y' - \tan \mathit{\Theta }}}{{1 + y'\tan \mathit{\Theta }}} $ | (39) |
将y′的表达式(37)代入(39):
| $ \begin{array}{l} \tan \alpha = \frac{{\frac{{r'\left( \mathit{\Theta } \right)\tan \mathit{\Theta } + r\left( \mathit{\Theta } \right)}}{{r'\left( \mathit{\Theta } \right) - r\left( \mathit{\Theta } \right)\tan \mathit{\Theta }}} - \tan \mathit{\Theta }}}{{1 + \frac{{r'\left( \mathit{\Theta } \right)\tan \mathit{\Theta } + r\left( \mathit{\Theta } \right)}}{{r'\left( \mathit{\Theta } \right) - r\left( \mathit{\Theta } \right)\tan \mathit{\Theta }}}\tan \mathit{\Theta }}}\\ = \frac{{r'\left( \mathit{\Theta } \right)\tan \mathit{\Theta } + r\left( \mathit{\Theta } \right) - r'\left( \mathit{\Theta } \right)\tan \mathit{\Theta } + r\left( \mathit{\Theta } \right){{\tan }^2}\mathit{\Theta }}}{{r'\left( \mathit{\Theta } \right) - r\left( \mathit{\Theta } \right)\tan \mathit{\Theta + }r'\left( \mathit{\Theta } \right){{\tan }^2}\mathit{\Theta } + r\left( \mathit{\Theta } \right)\tan \mathit{\Theta }}}\\ = \frac{{r\left( \mathit{\Theta } \right)}}{{r'\left( \mathit{\Theta } \right)}} \end{array} $ | (40) |
即
| $ \cot \alpha = \ln r\left( \mathit{\Theta } \right) + c = \ln \sqrt {{x^2} + {y^2}} + c $ | (41) |
取y=0为初始点(图中B点),此时Θ=0,代入式(41)则:
| $ c = - \ln {x_0} $ | (42) |
将式(42)代入式(41)得:
| $ \cot \alpha \cdot \mathit{\Theta } = \ln \frac{{r\left( \mathit{\Theta } \right)}}{{{x_0}}} $ | (43) |
因此该曲线极坐标方程为:r(Θ)=x0ecotα·Θ,是形如r=aekθ的对数螺旋线。
等螺距圆锥螺旋线在展开平面上的曲线方程为:r=x0Θ[2],是形如r=aθ的阿基米德螺线。
阿基米德螺线上A1, A2, A3, A4间的距离相等,如图 5所示。
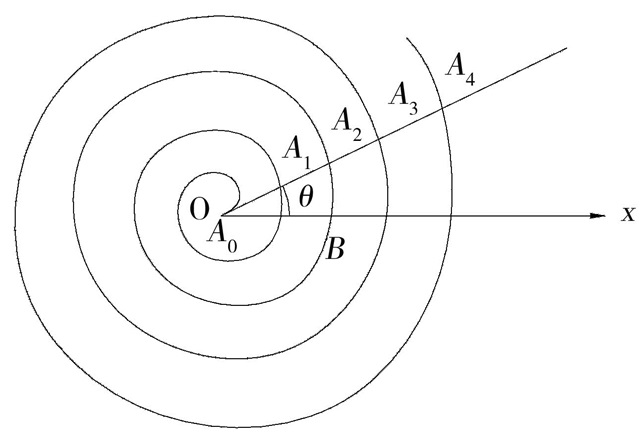
|
| 图 5 阿基米德螺线 Figure 5 Archimedes spiral |
等螺旋角及等螺距圆锥螺旋线不是圆锥面上的短程线,因为圆锥侧面展开之后这两条曲线分别是对数螺线和阿基米德螺线,不是直线。
由于圆锥侧面可展开为扇形,等螺旋角及等螺距圆锥螺旋线在圆锥侧面展开之后分别是对数螺线和阿基米德螺线,对于脱模困难而网格尺寸精度要求不高的双锥等结构,可将锥段阳模展开成平面,平面浇注后,再贴合到芯模上形成锥面螺旋筋槽,应注意阿基米德螺线的加工易于对数螺线。
为便于锥段复合材料网格结构阳模成型装置的设计生产和纤维缠绕,需要求出对数螺线和阿基米德螺线的弧长和曲率。
对数螺线r(Θ)=x0ecotα·Θ(-∞ < Θ < +∞)从矢径Θ=Θ1到矢径Θ=Θ2的弧长。
| $ \begin{array}{*{20}{c}} {S = \int_{{\mathit{\Theta }_1}}^{{\mathit{\Theta }_2}} {\sqrt {{r^2} + {{r'}^2}} {\rm{d}}\mathit{\Theta }} = {x_0}\sqrt {1 + {{\cot }^2}\alpha } \int_{{\mathit{\Theta }_1}}^{{\mathit{\Theta }_2}} {{{\rm{e}}^{\cot \alpha \cdot \mathit{\Theta }}}{\rm{d}}\mathit{\Theta }} }\\ { = \frac{{{x_0}\sqrt {1 + {{\cot }^2}\alpha } }}{{\cot \alpha }}\left( {{{\rm{e}}^{\cot \alpha \cdot {\mathit{\Theta }_2}}} - {{\rm{e}}^{\cot \alpha \cdot {\mathit{\Theta }_1}}}} \right)}\\ { = \frac{{{x_0}}}{{\cos \alpha }}\left( {{{\rm{e}}^{\frac{{{\mathit{\Theta }_2}}}{{\tan \alpha }}}} - {{\rm{e}}^{\frac{{{\mathit{\Theta }_1}}}{{\tan \alpha }}}}} \right)} \end{array} $ | (44) |
阿基米德螺线r=x0Θ(-∞ < Θ < +∞)从矢径Θ=Θ1到矢径Θ=Θ2的弧长。
| $ S = \int_{{\mathit{\Theta }_1}}^{{\mathit{\Theta }_2}} {\sqrt {{r^2} + {{r'}^2}} {\rm{d}}\mathit{\Theta }} = \int_{{\mathit{\Theta }_1}}^{{\mathit{\Theta }_2}} {\sqrt {{{\left( {{x_0}\mathit{\Theta }} \right)}^2} + x_0^2} {\rm{d}}\mathit{\Theta }} $ |
做双曲代换Θ=sht,dΘ=chtdt,t=arshΘ,
| $ \begin{array}{*{20}{c}} {S = {x_0}\int_{{\rm{arsh}}{\mathit{\Theta }_1}}^{{\rm{arsh}}{\mathit{\Theta }_2}} {{\rm{c}}{{\rm{h}}^2}t{\rm{d}}t} = {x_0}\int_{{\rm{arsh}}{\mathit{\Theta }_1}}^{{\rm{arsh}}{\mathit{\Theta }_2}} {\frac{{{\rm{ch}}2t + 1}}{2}{\rm{d}}t} }\\ { = \frac{{{x_0}}}{2}\left( {\frac{{{\rm{sh}}2t}}{2} + t} \right)/_{{\rm{arsh}}{\mathit{\Theta }_1}}^{{\rm{arsh}}{\mathit{\Theta }_2}} = \frac{{{x_0}}}{2}\left( {{\rm{sh}}t{\rm{ch}}t + t} \right)/_{{\rm{arsh}}{\mathit{\Theta }_1}}^{{\rm{arsh}}{\mathit{\Theta }_2}}}\\ { = \frac{{{x_0}}}{2}\left( {{\mathit{\Theta }_2}\sqrt {\mathit{\Theta }_2^2 + 1} - {\mathit{\Theta }_1}\sqrt {\mathit{\Theta }_1^2 + 1} + {\rm{arsh}}{\mathit{\Theta }_2} - {\rm{arsh}}{\mathit{\Theta }_1}} \right)} \end{array} $ | (45) |
对比展开前和展开后可知,Θ=sinβ·θ,
| $ {x_0} = \frac{a}{{\sin \beta }},螺距 z= 2\pi a\cos \beta ,a = \frac{z}{{2\pi \cos \beta }} $ |
| $ {x_0} = \frac{z}{{2\pi \cos \beta {\rm{sin}}\beta }} = \frac{z}{{\pi \sin 2\beta }} $ | (46) |
对数螺线的曲率:
| $ \left\{ \begin{array}{l} x = {x_0}{{\rm{e}}^{\cot \alpha \cdot \mathit{\Theta }}}\cos \mathit{\Theta }\\ y = {x_0}{{\rm{e}}^{\cot \alpha \cdot \mathit{\Theta }}}\sin \mathit{\Theta } \end{array} \right. $ | (47) |
| $ \left( \begin{array}{l} \frac{{{\rm{d}}x}}{{{\rm{d}}\mathit{\Theta }}} = {x_0}{{\rm{e}}^{\cot \alpha \cdot \mathit{\Theta }}}\left( {\cot \alpha \cos \mathit{\Theta } - \sin \mathit{\Theta }} \right)\\ \frac{{{\rm{d}}y}}{{{\rm{d}}\mathit{\Theta }}} = {x_0}{{\rm{e}}^{\cot \alpha \cdot \mathit{\Theta }}}\left( {\cot \alpha \sin \mathit{\Theta } + \cos \mathit{\Theta }} \right) \end{array} \right. $ | (48) |
| $ \left( \begin{array}{l} \frac{{{{\rm{d}}^2}x}}{{{\rm{d}}{\mathit{\Theta }^2}}} = {x_0}{{\rm{e}}^{\cot \alpha \cdot \mathit{\Theta }}}\left( {{{\cot }^2}\alpha \cos \mathit{\Theta } - 2\cot \alpha \sin \mathit{\Theta } - \cos \mathit{\Theta }} \right)\\ \frac{{{{\rm{d}}^2}y}}{{{\rm{d}}{\mathit{\Theta }^2}}} = {x_0}{{\rm{e}}^{\cot \alpha \cdot \mathit{\Theta }}}\left( {{{\cot }^2}\alpha \sin \mathit{\Theta } + 2\cot \alpha \cos \mathit{\Theta } - \sin \mathit{\Theta }} \right) \end{array} \right. $ | (49) |
曲率[4]:
| $ K = \frac{{\left| {\frac{{{\rm{d}}x}}{{{\rm{d}}\mathit{\Theta }}} \cdot \frac{{{{\rm{d}}^2}y}}{{{\rm{d}}{\mathit{\Theta }^2}}} - \frac{{{{\rm{d}}^2}x}}{{{\rm{d}}{\mathit{\Theta }^2}}} \cdot \frac{{{\rm{d}}y}}{{{\rm{d}}\mathit{\Theta }}}} \right|}}{{{{\left[ {{{\left( {\frac{{{\rm{d}}x}}{{{\rm{d}}\mathit{\Theta }}}} \right)}^2} + {{\left( {\frac{{{\rm{d}}y}}{{{\rm{d}}\mathit{\Theta }}}} \right)}^2}} \right]}^{\frac{3}{2}}}}} = \frac{{\sin \alpha }}{{{x_0}{{\rm{e}}^{\cot \alpha \cdot \mathit{\Theta }}}}} $ | (50) |
曲率半径:
| $ R = \frac{{{x_0}{{\rm{e}}^{\cot \alpha \cdot \mathit{\Theta }}}}}{{\sin \alpha }} $ | (51) |
阿基米德螺线的曲率:
| $ \left\{ \begin{array}{l} x = {x_0}\mathit{\Theta }\cos \mathit{\Theta }\\ y = {x_0}\mathit{\Theta }\sin \mathit{\Theta } \end{array} \right. $ | (52) |
| $ \left\{ \begin{array}{l} \frac{{{\rm{d}}x}}{{{\rm{d}}\mathit{\Theta }}} = {x_0}\cos \mathit{\Theta } - {x_0}\mathit{\Theta }\sin \mathit{\Theta }\\ \frac{{{\rm{d}}y}}{{{\rm{d}}\mathit{\Theta }}} = {x_0}\sin \mathit{\Theta } + {x_0}\mathit{\Theta }\cos \mathit{\Theta } \end{array} \right. $ | (53) |
| $ \left\{ \begin{array}{l} \frac{{{{\rm{d}}^2}x}}{{{\rm{d}}{\mathit{\Theta }^2}}} = - 2{x_0}\sin \mathit{\Theta } - {x_0}\mathit{\Theta }\cos \mathit{\Theta }\\ \frac{{{{\rm{d}}^2}y}}{{{\rm{d}}{\mathit{\Theta }^2}}} = 2{x_0}\cos \mathit{\Theta } - {x_0}\mathit{\Theta }\sin \mathit{\Theta } \end{array} \right. $ | (54) |
曲率:
| $ K = \frac{{2 + {\mathit{\Theta }^2}}}{{{x_0}{{\left( {1 + {\mathit{\Theta }^2}} \right)}^{\frac{3}{2}}}}} $ | (55) |
曲率半径:
| $ R = \frac{{{x_0}{{\left( {1 + {\mathit{\Theta }^2}} \right)}^{\frac{3}{2}}}}}{{2 + {\mathit{\Theta }^2}}} $ | (56) |
利用两点之间线段最短来求解圆锥侧面上的短程线,如图 6所示。设圆锥高为h,圆锥面方程:
x2+y2=(z-h)2tan2β,r=htanβ,A(r, 0, 0),B(x0, 0, z0),0<z0<h。设B在xOz的坐标为(xB, zB),求xB,zB。

|
| 图 6 圆锥面短程线的展开 Figure 6 Expansion of the cone geodesic line |
| $ \overrightarrow {OB} = \left( {\frac{{h - {z_0}}}{{\cos \beta }}\sin \left( {\beta + {\rm{ \mathit{ π} sin}}\beta } \right),h - \frac{{h - {z_0}}}{{\cos \beta }}\cos \left( {\beta + {\rm{ \mathit{ π} sin}}\beta } \right)} \right) $ | (57) |
| $ {x_{\rm{B}}} = \frac{{h - {z_0}}}{{\cos \beta }}\sin \left( {\beta + {\rm{ \mathit{ π} sin}}\beta } \right) $ | (58) |
| $ {z_{\rm{B}}} = h - \frac{{h - {z_0}}}{{\cos \beta }}\cos \left( {\beta + {\rm{ \mathit{ π} sin}}\beta } \right) $ | (59) |
从而在xOz上线段AB的方程为:
| $ \left\{ \begin{array}{l} x = \left( {{x_{\rm{B}}} - r} \right)t + r,\\ z = {z_{\rm{B}}}t, \end{array} \right.t \in \left[ {0,1} \right] $ | (60) |
在线段AB上任取一点N′(x′, z′),则当展开面重又绕回到锥面上时,其对应点N的坐标设为(x, y, z),求N点坐标,如图 7所示。
| $ \left| {MN} \right| = \left| {MN'} \right|,\angle AMN' = \varphi = \theta \cdot \sin \beta $ |
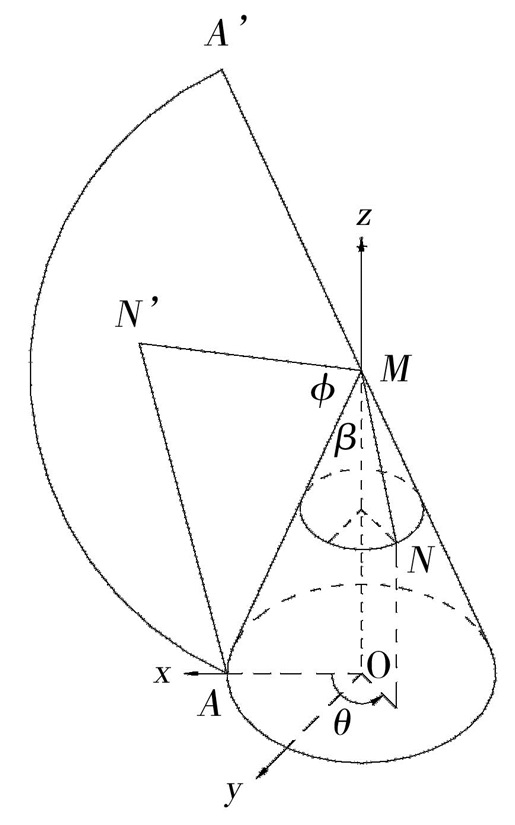
|
| 图 7 圆锥面短程线 Figure 7 Geodesic line of cone |
[与前述(46)式Θ=sinβ·θ结论相同]
| $ \therefore \left\{ \begin{array}{l} x = \left| {MN} \right|\sin \beta \cos \theta \\ y = \left| {MN} \right|\sin \beta \sin \theta \\ z = h - \left| {MN} \right|\cos \beta \end{array} \right. $ | (61) |
| $ \begin{array}{*{20}{c}} {\because \overrightarrow {ON'} = \overrightarrow {OM} + \overrightarrow {MN'} = \left( {0,h} \right) + \left| {MN'} \right|\left( {\sin \left( {\varphi + \beta } \right),} \right.} \\ {\left. { - \cos \left( {\varphi + \beta } \right)} \right)} \end{array} $ | (62) |
| $ \therefore \overrightarrow {ON'} = \left( {\left| {MN'} \right|\sin \left( {\varphi + \beta } \right),h - \left| {MN'} \right|\cos \left( {\varphi + \beta } \right)} \right) $ | (63) |
∵N′在AB上,
| $ \therefore \frac{{h - \left| {MN'} \right|\cos \left( {\varphi + \beta } \right)}}{{{z_{\rm{B}}}}} = \frac{{\left| {MN'} \right|\sin \left( {\varphi + \beta } \right) - r}}{{{x_{\rm{B}}} - r}} $ | (64) |
| $ \therefore \left| {MN'} \right| = \frac{{h\left( {{x_{\rm{B}}} - r} \right) + {z_{\rm{B}}}r}}{{{z_{\rm{B}}}\sin \left( {\varphi + \beta } \right) + \left( {{x_{\rm{B}}} - r} \right)\cos \left( {\varphi + \beta } \right)}} $ | (65) |
| $ \therefore \left\{ \begin{array}{l} x = \frac{{h\left( {{x_{\rm{B}}} - r} \right) + {z_{\rm{B}}}r}}{{{z_{\rm{B}}}\sin \left( {\varphi + \beta } \right) + \left( {{x_{\rm{B}}} - r} \right)\cos \left( {\varphi + \beta } \right)}}\sin \beta \cos \theta \\ y = \frac{{h\left( {{x_{\rm{B}}} - r} \right) + {z_{\rm{B}}}r}}{{{z_{\rm{B}}}\sin \left( {\varphi + \beta } \right) + \left( {{x_{\rm{B}}} - r} \right)\cos \left( {\varphi + \beta } \right)}}\sin \beta \sin \theta \\ z = h - \frac{{h\left( {{x_{\rm{B}}} - r} \right) + {z_{\rm{B}}}r}}{{{z_{\rm{B}}}\sin \left( {\varphi + \beta } \right) + \left( {{x_{\rm{B}}} - r} \right)\cos \left( {\varphi + \beta } \right)}}\cos \beta \end{array} \right. $ | (66) |
再将式(58)、(59)中xB,zB带入,即得到最终解。方程非常烦琐,不适合用于筋条的缠绕。但蒙皮的缠绕,纤维丝束在张力作用下是沿此方程缠绕的,即圆锥面蒙皮的缠绕其缠绕角度只能是近似按设计规定进行。在半锥角较小的情况下,此偏差影响不大[5]。缠绕时要注意对纤维束起点位置的调整。
另外,锥面短程螺旋线对于平面浇注软模成型的复合材料网格结构极具应用价值。在浇注阳模的模具上按图 4所示加工与扇形径向线夹角为α的直线筋槽即可。这将大大降低加工难度,提高加工效率,降低成本。为此计算直线与对数螺线的曲率差,由公式(43)r(Θ)=x0ecotα·Θ,结合工程应用中的小直径锥段,取α=30°,x0=1244.7。根据公式(50),θ=0°时,k得最大值为0.0004017,即曲率很小,可用直线代替对数螺线,不影响结构承载。对于大直径锥段,曲率更小,更接近直线。
3 结论通过对圆柱、圆锥侧面上的螺旋线和短程线的探讨,解决了圆柱、圆锥段复合材料网格结构筋条及蒙皮的缠绕问题;得出了圆柱和锥段螺旋线的弧长和曲率计算方法,用于圆柱段和圆锥段复合材料网格结构成型装置的设计生产和纤维缠绕;得出了对数螺线和阿基米德螺线的弧长和曲率计算方法,用于锥段复合材料网格结构软模成型装置的设计生产和纤维缠绕。为复合材料网格结构自动化缠绕生产制造创造了条件。同时针对工程需要,对不同的网格结构选择不同的加工成型方式,可大大降低加工难度,实现低成本快速批量生产。
| [1] | 牛春匀. 实用飞机复合材料结构设计与制造[M]. 北京: 航空工业出版社, 2010: 176. |
| [2] | 王连祥, 方德植, 等. 数学手册[M]. 北京: 人民教育出版社, 1979: 409, 411-412. |
| [3] |
提亚峰, 张铎, 董波. C/E复合材料网格缠绕结构一体化设计[J].
宇航材料工艺, 2010, 40(6): 33–37.
|
| Cited By in Cnki | |
| [4] | 同济大学数学教研室主编. 高等数学[M]. 第三版, 上册. 北京: 高等教育出版社, 1988: 217. |
| [5] |
提亚峰. 复合材料网格结构参数调整及优化计算[J].
宇航系统工程, 2013, 3(3): 26–34.
|


